Introduction to Finance: Class 1

Time Value of Money

What is the time value of money?

Simply put, the value of money is dependent upon time. The purchasing power of money varies with time. 

Time value of money is a fundamental financial principle that asserts that money now is worth more than money received in the future.

—Source

Although it may be a simple concept, it has much magnitude, being applicable to all financial aspects of life. This principle lies as the foundation for finance and answers the question, “What if?” when it comes to making financial decisions.

Time Value of Money Video

The time value of money is broken down into four key concepts…
Key Concepts:

• Future Value
• Present Value
• Discount Rates
• Number of Periods

Future Value

The time value of money tells us what the present value of an investment will grow to by a given date. This is its future value. The difference between the present value and the future value depends on how many compounding periods are involved in the investment, and on the interest rate.

—Source

Future Value: One-Period Example

• Suppose you invest $100 in a savings account that pays 10% interest per year. How much will you have in one year?
  • Interest = $100 x 0.10 = $10
  • Value in one year = principal + interest
  • Value in one year = $100 + $10
  • Value in one year = $110
  • Future Value (FV) = $100 x (1 + 0.10) = $110

Future Value: Two-Period Example

• Suppose you leave your $100 in the bank for two years and the interest rate remains 10%. How much will you have in two years?
  • Interest in year 2 = $110 x 0.10 = $11
  • Value in two years = principal + interest yr 1 + interest yr 2
  • Value in one year = $100 + $10 + $11
  • Value in one year = $121

• $121 is the FV of $100 in two years at 10%
  • $121 = $110 x (1 + 0.10)
  • $121 = [$100 x (1 + 0.10)] x (1 + 0.10)
  • $121 = $100 x (1 + 0.10)²

Future Value: General Result

• How much will you have in three years at 10%?
  • $121 x (1 + 0.10) = $133.1
  • [$100 x (1 + 0.10)²] x (1 + 0.10) = $133.1
  • $100 x (1 + 0.10)³ = $133.1

• General Result
  • Future Value = $ Invested x (1 + r)^t
    • r = one-period interest rate (expressed as decimal point)
    • t = number of periods invested
    • (1 + r)^t = Future value interest factor – FVIF(r,t)

 · · · · ·

Simple and Compound Interest

• Simple interest: interest earned on original investment
• Compound interest: interest earned on interest reinvested

Closer look at our two-period example:

$121 FV has four components

• $100 original investment/principal
• $10 simple interest in year 1
• $10 simple interest in year 2
• $1 compound interest in year 2

• Over the two-year period, you earn $20 in simple interest and $1 in compound interest

 · · · · ·

• Suppose 200 years ago your relative deposited $10 in a bank account earning an annual interest of 5.5%. How much would the investment be worth today?
• What portion of the future value of your investment comes from:
  • Original principal
  • Simple interest  
  • Compound interest  

• A related question is: How much would we need our relatives to invest 200 years ago at 5.5% per year in order for us to receive $1M today?
  • To answer this question, we need to know how to calculate a present value

Present Value

The current worth of a future sum of money or stream of cash flows given a specified rate of return. Future cash flows are discounted at the discount rate, and the higher the discount rate, the lower the present value of the future cash flows. Determining the appropriate discount rate is the key to properly valuing future cash flows, whether they be earnings or obligations.

—Source

Present Value: One-Period Example

• Suppose you need $10,000 in one year for the down payment on a new car. If your bank account pays a 7% annual interest rate, how much do you need to save today?
  • FV = $ Invested x (1 + r)
  • $ Invested = FV / (1 + r)
  • $ Invested = $10,000 / (1 + 0.07)
  • $ Invested = $9,345.79

• “$ Invested” = Present Value (PV) of investment
• The PV of $10,000 discounted one year at 7% is $9,345.79

Present Value: General Result

• Rearrange Future Value Formula:
  • FV = PV x (1 + r)^t      →     PV = FV / (1 + r)^t

• How much do we save today if we need the $10K down payment in two years and the discount rate is still 7%?
  • PV = $10,000 / (1 + 0.07)²
  • PV = $8,734.39

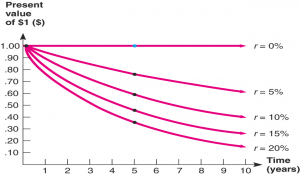
Present Value: Two Important Relationships

1. For a given interest rate – the longer the time period, the lower the present value (all else equal)
   • What is the present value of $500 to be received in 5 years? 10 years? The discount rate is 10%
     • 5 Years: 0PV = $500 / (1 + 0.10)⁵ = $310.46
     • 10 Years: PV = $500 / (1 + 0.10)¹⁰ = $192.77

2. For a given time period – the higher the interest rate, the lower the present value (all else equal)
   • What is present value of $500 received in 5 years if the interest rate is 10%? 15%?
     • Rate = 10%: PV = $500 / (1 + 0.10)⁵ = $310.46
     • Rate = 15%: PV = $500 / (1 + 0.15)⁵ = $248.59

 · · · · ·

• So, how much would we need our relatives to invest 200 years ago at 5.5% per year in order for us to receive $1M today?
• If the interest rate was only 3% per year, then how much would they need to invest for us to receive $1M?

• A related example: Now, our relatives only have $5 to invest 200 years ago. What interest rate must the bank pay for our family bank account to be worth $1M today?
  • To answer this question, we need to know how to calculate the discount rate

Discount Rate

The discount rate also refers to the interest rate used in discounted cash flow (DCF) analysis to determine the present value of future cash flows. The discount rate in DCF analysis takes into account not just the time value of money, but also the risk or uncertainty of future cash flows; the greater the uncertainty of future cash flows, the higher the discount rate.

—Source

Discount Rate Video

Discount Rate

• Rearrange PV equation and solve for r
  • FV = PV x (1 + r)^t      →     r = (FV/PV)^1/t – 1

Discount Rate: Example

• You are looking at an investment that will pay $1,200 in 5 years if you invest $1,000 today.  What is the implied rate of interest?
  • r = ($1,200 / $1,000)^1/5 – 1 = .03714 = 3.714%

 · · · · ·

• What interest rate must we earn for our account to be worth $1M today if your relative deposited $5 in the bank 200 years ago?
• What interest rate do we need if they only saved $1?

Number of Periods

If we know the present value (PV), the future value (FV), and the interest rate per period of compounding (r), the future value factors allow us to calculate the unknown number of time periods of compound interest (t). 

—Source

Finding the Number of Periods

• Finally, our relative had exactly $3 to save for us and found a bank account that paid 5% for as long as it is open.  How long will our family have to wait until the account is worth $1M?
• Start with basic equation and solve for t
  • FV = PV(1 + r)^t
  • t = ln(FV / PV) / ln(1 + r)

Number of Periods: Example

• You want to purchase a new car and you are willing to pay $20,000. If you can invest at 10% per year and you currently have $15,000, how long will it be before you have enough money to pay cash for the car?
• t = ln($20,000 / $15,000) / ln(1.1) = 3.02 years

 · · · · ·

• How many years will our family have to wait for our bank account to grow to $1M if our relative originally invested $3 and the annual interest rate is 5%?
• How long will it take to grow to $2M?

Wrap-Up

• Basic Present Value Equation: PV x (1 + r)^t = FV
• Given any three pieces of information in the equation, you can solve for the fourth

• PV = FV / (1 + r)^t
• r = (FV/PV)^1/t – 1
• t = ln(FV / PV) / ln(1 + r)

• More learning tools:
  • This overview on Study Finance covers an introduction to simple interest and compound interest, illustrates the use of time value of money tables, shows a matrix approach to solving time value of money problems, and introduces the concepts of intrayear compounding, annuities due, and perpetuities. A simple introduction to working time value of money problems on a financial calculator is included as well as additional resources to help understand time value of money.
  • Khan Academy provides an insightful perspective on the time value of money through video instruction. It’s a great source for those visual learners!
  • Teenvestor provides further explanation on the concept.
  • Accounting Coach also provides in-depth explanations and examples that can be useful to further one’s understanding.

 · · · · ·

Solutions

1.

• Suppose you had a relative deposit $10 at 5.5% interest 200 years ago. How much would the investment be worth today?
  • FV = $10 x (1 + 0.055)²⁰⁰ = $447,189.84

• What is the effect of compounding?
  • Principal = $10
  • Simple interest = ($10 x .055) x 200 = $110
  • Compound interest = $447,189.84 – $10 – $110
  • Compound interest = $447,069.84    

2.

• So, how much would we need our relatives to invest 200 years ago at 5.5% per year for us to receive $1M today?
  • PV = FV / (1 + r)^t             PV = $1M / (1 + 0.055)²⁰⁰             PV = $22.36

• If the interest rate was only 3% per year, then how much would they need to invest for us to receive $1M?
  • 200 N
  • 3 I/Y
  • 1,000,000 FV
  • (CPT) PV = -$2,707.42  

 3.

• What interest rate must we earn for our account to be worth $1M today if your relative deposited $5 in the bank 200 years ago?
  • r = ($1M / $5)^1/200 – 1 = 0.0629 → 6.29%

• What interest rate do we need if they only saved $1?
  • 200 N
  • -1 PV
  • 1,000,000 FV
  • (CPT) I/Y = 7.15  

4.

• How many years will our family have to wait for our bank account to grow to $1M if our relative originally invested $3 and the annual interest rate is 5%?
  • t = ln($1M / $3) / ln(1 + 0.05) = 260.64 years

• How long will it take to grow to $2M?
  • 5 I/Y
  • -3 PV
  • 2,000,000 FV
  • (CPT) N = 274.85      

For more classes:

Education Series